Number Structure II 1984
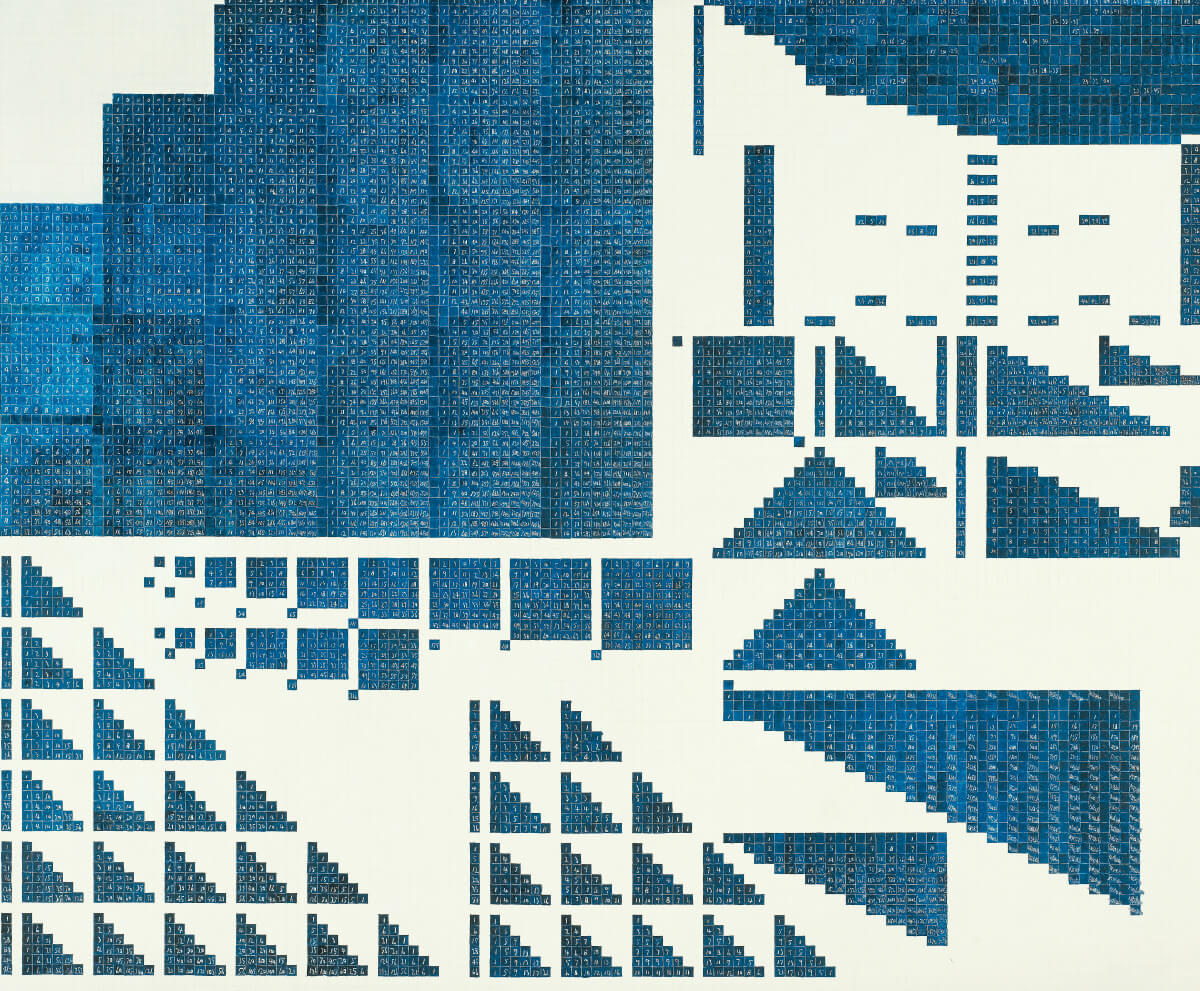
Kazuo Nakamura, Number Structure II, 1984
Oil and graphite on canvas, 127 x 152.3 cm
Art Gallery of Ontario, Toronto
Kazuo Nakamura dedicated the last decade of his career to studying number progressions and patterns, the foundation that underlies the universe and all of nature. Number Structure II is the acme of that work. The painting is made up of minute blue squares—most inscribed with numbers—arranged in patterns on an ivory ground. The specific mathematical functions referenced in this painting include Sierpinski triangles, Pascal’s triangles, Catalan numbers, and magic squares. It is likely the last painting he made, except for a few variants of the early 1960s landscapes that were popular with collectors and sold well.


Number Structure II is part of a series of works that illustrate numerical progressions such as Fibonacci numbers, or more generally numerical combinations like magic squares, as illustrated in Untitled (Magic Squares), c.1975–85. They are hand-painted, most often using blue and white, which recalls yet again the aizuri-e, Japanese woodcut prints. The use of mathematics and the grid patterns they generate contrast with the numbers, all written out by hand and echoing the hand-drawn lines in the Minimalist works of Agnes Martin (1912–2004). The paintings are yet another encounter between the human mind, expressed by the handwriting, and the universe the mind seeks to describe, expressed by numbers. Here that dialogue is reduced to something of its essence through numerical patterns that exist in nature.
What is interesting in Nakamura’s study of these numerical sequences is that they focus on patterns as opposed to theorems, which describe the behaviours of natural phenomena. The distinction between the two is not necessarily huge, but patterns have an aesthetic quality that would attract the eye of an artist. Nakamura was certainly thinking more broadly. He said, “I was always interested in internal structures, the law of order that lies in everything,” but ultimately he focused single-mindedly on numerical patterns.
What triggered this fascination—some would say obsession—may have been the “discovery” of fractals by Benoit Mandelbrot. In 1975, the mathematician published a paper in which he coined the term “fractal” to describe a form of geometric repetition in which a rough shape can be divided into parts, each of which is a progressively smaller copy of the same overall pattern—think of ferns or snowflakes. Nakamura started working out numerical progressions on reams and reams of graph paper around the same time. It became, as he stated in 1993, “my most important work now.” And art dealer Christopher Cutts noted after Nakamura’s death in 2002 that “Kaz was looking for the Grand Theory…. This search was his holy grail, his chalice. He felt number-structure works constituted his legacy.”
Although his work with number sequences never ended, as testified by the bundles of progressions on paper Nakamura produced up until his death, he did abandon painting with Number Structure II. As he stated in an interview: “I’d like to push myself as far as I can into the realm of the theoretical. When I work like this, I’m always discovering something new. Whereas painting is just translation.” He concluded: “I hope to go back to painting.” Ironically, when one could claim that Nakamura had reached the most abstract form of expression possible, he himself felt the contrary: he was exposing great universal truths. He declared, “You might just say that I am actually a realist.”

 About the Author
About the Author
 More Online Art Books
More Online Art Books
 Acknowledgements
Acknowledgements